Section 2 6 Geometric Proof

Section 2 6 geometric proof – Welcome to the fascinating realm of geometric proofs, where Section 2.6 emerges as a cornerstone of mathematical precision. In this section, we embark on an adventure to uncover the secrets of congruence, similarity, angle bisectors, and perpendicular bisectors. Brace yourself for a journey that will sharpen your geometric intuition and equip you with the tools to conquer any geometric challenge.

Section 2.6 unveils a treasure trove of theorems and properties that empower us to establish the equality or similarity of geometric figures. We will explore the conditions that govern the congruence and similarity of triangles, unlocking the secrets of these fundamental geometric shapes.

Introduction

Section 2.6 plays a pivotal role in geometric proofs by providing a systematic approach to establish the congruence and similarity of geometric figures. This section delves into fundamental concepts such as congruence, similarity, and transformations, equipping students with the tools to construct logical arguments and solve geometric problems effectively.

The key concepts covered in this section include:

Congruence: Establishing that two figures have the same shape and size.
Similarity: Determining that two figures have the same shape but may differ in size.
Transformations: Exploring geometric operations like translations, rotations, and reflections that preserve congruence or similarity.
Proofs using transformations: Utilizing transformations to prove congruence or similarity between figures.

Congruence

Congruence is a fundamental concept in geometry that establishes the equivalence of two figures in terms of both shape and size. Two figures are considered congruent if they have the same shape and their corresponding sides and angles are equal in measure.

Congruence is often represented using the symbol ≅.

Proving congruence involves demonstrating that corresponding sides and angles of two figures are equal. This can be achieved through various methods, including:

Using properties of congruent triangles (e.g., SSS, SAS, ASA, AAS).
Applying transformations to establish congruence (e.g., rotations, reflections).
Utilizing indirect proofs to eliminate possibilities and arrive at the conclusion of congruence.

Congruence Theorems

Congruence theorems are a set of rules that allow us to determine whether two triangles are congruent. In other words, they tell us when two triangles have the same shape and size. There are several different congruence theorems, each of which is based on a different set of conditions.

The most basic congruence theorem is the Side-Side-Side (SSS) theorem. This theorem states that if two triangles have three pairs of congruent sides, then the triangles are congruent.

Another common congruence theorem is the Side-Angle-Side (SAS) theorem. This theorem states that if two triangles have two pairs of congruent sides and a pair of congruent angles included between those sides, then the triangles are congruent.

The Angle-Side-Angle (ASA) theorem is similar to the SAS theorem, but it uses two pairs of congruent angles and a pair of congruent sides instead. The Hypotenuse-Leg (HL) theorem is used to prove congruence of right triangles.

SSS Theorem

The SSS theorem is one of the most straightforward congruence theorems. It states that if three sides of one triangle are congruent to three sides of another triangle, then the triangles are congruent.

SSS Theorem: If $\triangle ABC \cong \triangle DEF$, then $AB = DE$, $BC = EF$, and $AC = DF$.

This theorem can be used to prove congruence of triangles in a variety of situations. For example, it can be used to prove that two triangles are congruent if they have the same base and the same height.

SAS Theorem

The SAS theorem is another common congruence theorem. It states that if two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, then the triangles are congruent.

SAS Theorem: If $\triangle ABC \cong \triangle DEF$, then $AB = DE$, $BC = EF$, and $\angle B = \angle E$.

ASA Theorem

The ASA theorem is similar to the SAS theorem, but it uses two pairs of congruent angles and a pair of congruent sides instead.

ASA Theorem: If $\triangle ABC \cong \triangle DEF$, then $\angle A = \angle D$, $\angle B = \angle E$, and $AB = DE$.

HL Theorem

The HL theorem is used to prove congruence of right triangles.

HL Theorem: If $\triangle ABC$ and $\triangle DEF$ are right triangles, and if $AB = DE$ and $BC = EF$, then $\triangle ABC \cong \triangle DEF$.

This theorem can be used to prove congruence of right triangles in a variety of situations. For example, it can be used to prove that two right triangles are congruent if they have the same hypotenuse and one other side.

Similarity Theorems: Section 2 6 Geometric Proof

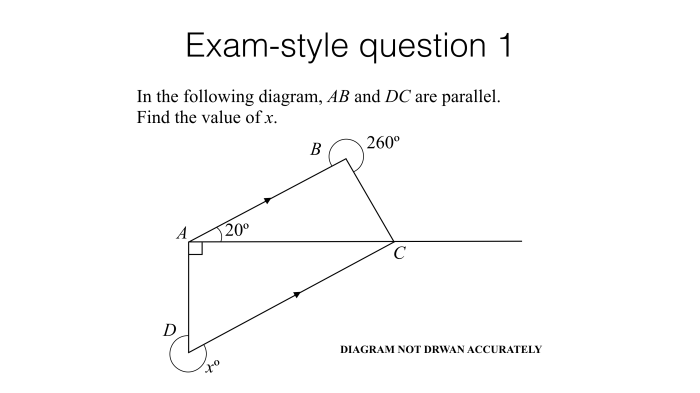
In geometry, similarity is a transformation that preserves the shape of a figure but not necessarily its size. Two figures are similar if they have the same shape but not necessarily the same size. Similarity theorems are used to prove that two triangles are similar.

There are three similarity theorems: the Side-Side-Side (SSS) Similarity Theorem, the Side-Angle-Side (SAS) Similarity Theorem, and the Angle-Side-Angle (ASA) Similarity Theorem.

SSS Similarity Theorem

The SSS Similarity Theorem states that if the three sides of one triangle are proportional to the three sides of another triangle, then the triangles are similar.

If $\triangle ABC \sim \triangle DEF$, then $\fracABDE = \fracBCEF = \fracACDF$.

SAS Similarity Theorem

The SAS Similarity Theorem states that if two sides of one triangle are proportional to two sides of another triangle, and the included angles are congruent, then the triangles are similar.

If $\triangle ABC \sim \triangle DEF$, then $\fracABDE = \fracBCEF$ and $\angle B \cong \angle E$.

ASA Similarity Theorem

The ASA Similarity Theorem states that if two angles of one triangle are congruent to two angles of another triangle, and the included sides are proportional, then the triangles are similar.

If $\triangle ABC \sim \triangle DEF$, then $\angle A \cong \angle D$, $\angle B \cong \angle E$, and $\fracABDE = \fracACDF$.

Section 2 6 geometric proof focuses on understanding the properties of geometric figures. A related concept is “ok to eat,” which can refer to the edibility of certain items. If you’re looking for a crossword clue related to this topic, check out ok to eat crossword clue . Returning to section 2 6 geometric proof, these principles are essential for solving complex geometric problems and comprehending spatial relationships.

Angle Bisector Theorem

The Angle Bisector Theorem states that if a line bisects an angle of a triangle, then it divides the opposite side into segments that are proportional to the lengths of the adjacent sides. In other words, if $m$, $n$, $p$, and $q$ are the lengths of the segments created by the angle bisector, then:$$\fracmn = \fracpq$$This theorem is useful for finding the lengths of sides in triangles and for solving geometry problems involving angle bisectors.

Properties of Triangles with Angle Bisectors

There are several properties of triangles with angle bisectors that can be derived from the Angle Bisector Theorem. These properties include:

The angle bisector of an angle of a triangle is also the perpendicular bisector of the opposite side.
The angle bisectors of the three angles of a triangle intersect at a single point, called the incenter of the triangle.
The incenter of a triangle is equidistant from the three sides of the triangle.

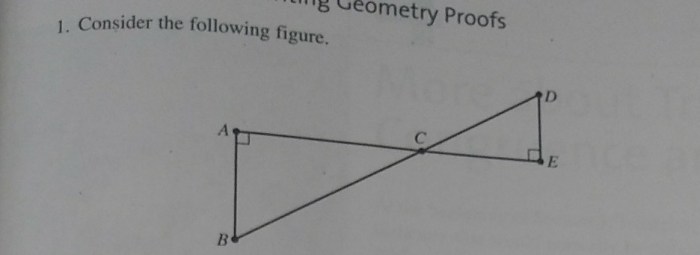
Geometric Proofs Involving the Angle Bisector Theorem, Section 2 6 geometric proof

There are several geometric proofs that involve the Angle Bisector Theorem. One of the most common proofs is the following:Proof:Let $ABC$ be a triangle with angle bisector $AD$. Let $m$, $n$, $p$, and $q$ be the lengths of the segments created by the angle bisector, as shown in the diagram below.[Image

of a triangle with angle bisector]By the Angle Bisector Theorem, we have:$$\fracmn = \fracpq$$We also have:$$m + n = p + q$$(since $AD$ is the bisector of angle $A$).Substituting the second equation into the first equation, we get:$$\fracmn = \fracpq = \fracm + np + q = \fracADBD$$Therefore, $AD : BD = m : n$.

Perpendicular Bisector Theorem

The Perpendicular Bisector Theorem states that the perpendicular bisector of a line segment is the set of all points equidistant from the endpoints of the segment.

In other words, if a line segment has a perpendicular bisector, then every point on the perpendicular bisector is the same distance from both endpoints of the line segment.

Properties of Perpendicular Bisectors

The perpendicular bisector of a line segment is a line that passes through the midpoint of the segment and is perpendicular to the segment.
Every point on the perpendicular bisector of a line segment is equidistant from the endpoints of the segment.
The perpendicular bisector of a line segment divides the segment into two congruent segments.

Geometric Proofs Using the Perpendicular Bisector Theorem

The Perpendicular Bisector Theorem can be used to prove a variety of geometric theorems. For example, it can be used to prove that the diagonals of a rectangle are congruent and that the opposite sides of a parallelogram are congruent.

Coordinate Geometry

Coordinate geometry plays a crucial role in Section 2.6 by providing a powerful tool for proving geometric properties. By assigning coordinates to points on a plane, we can use algebraic equations to describe geometric figures and relationships between them.

Examples of Geometric Proofs Involving Coordinate Geometry

One common application of coordinate geometry in geometric proofs is to prove that two figures are congruent. By showing that the coordinates of corresponding points in the figures are equal, we can establish their congruence. For instance, we can use the distance formula to prove that two triangles are congruent by showing that their corresponding sides have equal lengths.Another

application of coordinate geometry is to prove that two figures are similar. By showing that the ratios of the corresponding sides of the figures are equal, we can establish their similarity. For example, we can use the slope formula to prove that two triangles are similar by showing that their corresponding sides have the same slope.Coordinate

geometry also allows us to prove angle relationships. By using the dot product or cross product of vectors, we can determine whether two lines are perpendicular or parallel. For instance, we can use the dot product to prove that two lines are perpendicular by showing that their direction vectors are orthogonal.

Applications of Geometric Proofs

Geometric proofs are not just abstract mathematical exercises; they have practical applications in various fields, including architecture, engineering, and design.

In architecture, geometric proofs are used to ensure the structural integrity of buildings and bridges. Architects use geometric principles to calculate the forces acting on a structure and to design it in a way that can withstand those forces. For example, the ancient Greeks used geometric proofs to design the Parthenon, which has stood for over 2,000 years.

In engineering, geometric proofs are used to design machines, bridges, and other structures. Engineers use geometric principles to calculate the stresses and strains on a structure and to design it in a way that can withstand those forces. For example, engineers use geometric proofs to design airplanes, which must be able to withstand the forces of lift, drag, and gravity.

In design, geometric proofs are used to create aesthetically pleasing and functional objects. Designers use geometric principles to create objects that are both beautiful and practical. For example, graphic designers use geometric proofs to create logos and other visual designs.

deandre